Main page
Department of Mathematics and Statistics
of University of Helsinki
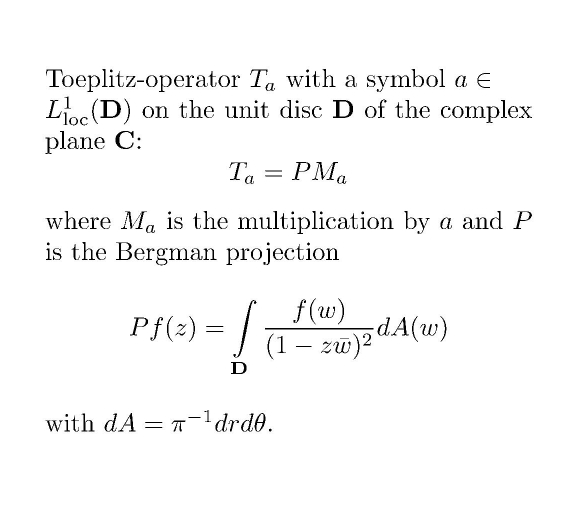
Formula 1. Toeplitz-operator and Bergman projection
|
Bergman space Ap with 1 ≤ p < ∞
is the closed
subspace of Lp consisting of analytic functions. We consider here the case
the domain is the open unit disc D of the complex plane
C and the Lp -space is defined
with the normalized two-dimensional
Lebesgue measure dA.
The Toeplitz-operator Ta is
defined as the
compose of the multiplication Ma and the Bergman projection
P . Obviously,
since P : Lp → Ap
is well defined and bounded in case 1 < p < ∞ ,
any bounded symbol a defines a bounded Toeplitz-operator
Ta : Ap → Ap . Nearly equally
obviously, very badly singular functions (indeed, distributions) which
are compactly supported in D, also define bounded Toeplitz-operators.
A satisfactory characterization of the boundedness of the operator
in terms of the symbol is a remarkable open problem.
SOME RECENT ARTICLES
-
J.Bonet, W.Lusky, J.Taskinen:
On boundedness and compactness of Toeplitz operators in weighted H^\infty-spaces.
To appear in J. Functional Anal.
This is a study of Toeplitz operators
with radial symbols on the unit disc in weighted H^\infty spaces.
Assuming that the weight satisfies the condition (B) introduced by the
second author, we are able to characterize the boundedness and compactness of
these operators. Note that the corresponding characterization remains
unknown in the Bergman spaces A^p with 1 < p < \infty, unless p = 2.
One of the starting points is that for radial symbols, the Toeplitz operators
are just Taylor coefficient multipliers, and this creates a link to our recent
studies on solid hulls, although the results cannot be directly drawn from them.
In addition, we formulate a related sufficient condition for the boundedness
in reflexive weighted Bergman spaces.
Moreover, it is known by results of M.Dostanic that the Bergman projection
may behave badly in weighted Bergman spaces with p not equal to 2, if the
weight is rapidly decreasing at the boundary of the disc. We complement this
result by constructing a bounded harmonic symbol such that the
corresponding Toeplitz operator is not bounded in any weighted H_v^\infty
space with the weight v satisfying some mild assumptions. As a corollary, the
Bergman projection is never bounded with respect to the corresponding
weighted sup-norms.
Preprint
-
A.Karapetyants, J.Taskinen:
Toeplitz operators with radial symbols on weighted holomorphic Orlicz spaces.
Submitted.
We consider a class of Toeplitz operators with radial symbols
on weighted holomorphic Orlicz space. Our result is a generalization of
that in the paper by W. Lusky and the second author in Studia Math.
204 (2011), 137-154 (see below): we characterize the boundedness and
compactness in the case some iterated radial integral of the symbol
is positive.
Preprint
-
J.Taskinen, J.Virtanen:
On compactness of Toeplitz operators in Bergman spaces.
Functiones Approximatio 59.2 (2018), 305-318.
We give a characterization of compact Toeplitz operators in Bergman spaces A^p, 1 < p < \infty,
of the unit disc with symbols in L^1 under a mild additional condition. Our result is new even in the
Hilbert space setting p=2, where it extends the well-known characterization of
compact Toeplitz operators with bounded symbols by Stroethoff and Zheng (Transactions AMS -92).
Preprint
-
J.Taskinen, J.Virtanen:
On generalized Toeplitz and little Hankel operators on Bergman spaces.
Archiv Math. 110, 2 (2018), 155--166.
This work complements the Rev.Mat.Iberoamericana-2010-paper of the authors (below).
We find a concrete integral formula for the class of generalized Toeplitz
operators, studied in the above citation. The result is extended to little Hankel operators.
We show that the approximating series and limits for the generalized Toeplitz operators converge in the strong operator topology.
We give an example of an L2-symbol a such that the Toeplitz operator with symbol
|a| fails to be bounded, although the operator with symbol a can be seen
seen to be bounded by using our generalized definition. We also confirm that the
generalized definition coincides with the classical one whenever the latter makes sense.
Preprint
-
A.Perälä, J.Taskinen, J.Virtanen:
Toeplitz operators on Dirichlet-Besov spaces.
Houston J. Math. 43, 1(2017), 93_-108.
We study Toeplitz operators Ta on the Besov spaces Bp
in the case of the open unit disk and finite p > 1 . We prove that a symbol a
satisfying a weak Lipschitz type condition induces a bounded operator Ta . Such symbols do not need to
be bounded functions or have continuous extensions to the boundary of unit disc. We discuss the problem of
the existence of nontrivial compact Toeplitz operators and also consider Fredholm properties and
prove an index formula.
Preprint
-
J.Bonet, J.Taskinen:
A note about Volterra operators on weighted Banach spaces of entire functions.
Math. Nachrichten 288 (2015), 1216-1225.
We characterize boundedness, compactness and weak compactness of Volterra operators
Vg acting between different
weighted Banach spaces H_v^\infty of entire functions with sup-norms in terms of the symbol g; thus we
complement recent work by Bassallote, Contreras, Hernáandez-Mancera, Martín and Paul for spaces
of holomorphic functions on the disc and by Constantin and Peláez for reflexive weighted Fock spaces.
Preprint
-
A.Perälä, J.Taskinen, J.Virtanen:
New results and open problems on Toeplitz operators in Bergman spaces.
New York J. Math. 17a (2011), 147-164.
We review recent developements of the theory of Toeplitz operators
in Bergman spaces. In addition we consider the special case of radial
distributional symbols on the disc. We reformulate the sufficient conditions
of the Rev.Math.Iberoam- and P.E.M.S.-papers (both below) for this case and
show that the latter condition is weaker than the former.
There is also a short remark on the possibility to extend the definition of
Toeplitz operators even beyond distributional symbols, i.e. to the case of
hyperfunctions.
Preprint
-
A.Perälä, J.Taskinen, J.Virtanen:
Toeplitz operators with distributional symbols
on Fock spaces.
Funct. et approx. 44,2 (2011), 203-213.
We show how Toeplitz operators with distributional symbols can be defined
and treated in Fock spaces of entire analytic functions. The approach is
a nontrivial adaptation of the P.E.M.S.-paper to the Fock space setting.
Preprint
-
W.Lusky, J.Taskinen:
Toeplitz operators on Bergman spaces and Hardy multipliers.
Studia Math. 204 (2011), 137-154.
The setting is Toeplitz operators Ta
in weighted Bergman spaces on the unit
disc; both the symbols and weights are assumed radial. In the Hilbert space
case Grudsky, Karapetyants and Vasilevski showed that the Toeplitz operator
is unitarily equivalent to the Taylor coefficient multiplier and used this
approach to derive a variety of results for Ta.
The same is not possible in the case of non-Hilbert Bergman spaces, since
the monomials do not form an unconditional Schauder bases there. However,
using the methods of the first named author, it is still possible to decompose
the Bergman space into a sequence of finite dimensional blocks. The
restriction of Ta onto these blocks can be still
be considered as a coefficient multiplier, and this way we can derive a
connection of the boundedness problem of Ta in the
Bergman space to the boundedness problem of Hardy multipliers.
In the other part of the paper we characterize the bounded
Toeplitz operators under an additional weak assumption on the positivity
of certain indefinite integrals of the symbol. This is a generalization of
the well-known result on boundedness for positive symbols.
Preprint
-
J.Taskinen, J.Virtanen:
Weighted BMO and Toeplitz operators on the Bergman space A¹.
J.Operator Th. 68,1 (2012), 131-140.
We adopt the results of the Rev.Math.Iberoam.-paper to the case
p=1. In addition to an inevitable logarithmic correction, it
is necessary to consider separately BA and BO type
symbols.
-
J.Taskinen, J.Virtanen:
Toeplitz operators on Bergman spaces with locally
integrable symbols.
Rev.Math.Iberoamericana. 26,2 (2010), 693-706.
For positive symbols the boundedness of Ta: Ap
→ Ap, 1 < p < ∞, can be
characterized
by the boundedness of averages of the symbol a over all hyperbolic discs
with some constant radius. It is quite obvious that the straightforward
generalization of this condition for nonpositive a (by replacing
a with |a|) is a sufficient but not necessary condition for
boundedness of Ta: Ap
→ Ap.
In the present work we give a much weaker sufficient condition, which
is still a kind of averaging condition over hyperbolic rectangles (however,
for a itself, rather than for |a|). We also
give an example of a symbol which satisfies our condition but is not
integrable over the unit disc.
We also consider sufficient compactness and Fredholmness conditions,
and prove a rather general index formula, which again uses an average
function instead of a usual pointwise condition for a.
-
A.Perälä,J.Taskinen, J.Virtanen:
Toeplitz operators with distributional symbols
on Bergman spaces.
Proc.Edinburgh Math.Soc. 54, 2 (2011), 505-514.
It is not at all difficult to define Toeplitz-operators with distributional
symbols for distributions which are compactly supported in D: the integral
formula defining a usual Toeplitz operator can be seen as a duality bracket of
a distribution and an infinitely smooth test function (which is the function
f ∈ Ap times the Bergman kernel, cf. Formula 1).
We give the definition of a general class of Toeplitz-operators with noncompactly
supported distributions as symbols. The operators are well defined and bounded
on the reflexive Bergman spaces, as long as the symbol belongs to a negative
order weighted Sobolev space, the weight being the boundary distance to the power
-α and α being the order of the distribution.
The corresponding condition for compactness is also provided.
-
J.Taskinen, J.Virtanen:
Spectral theory of Toeplitz and Hankel operators on the
Bergman space A¹.
New York J. Math. 14 (2008),1-19.
In case p=1 the Bergman projection is not bounded, contrary to the
reflexive cases. Consequently, the conditions sufficent for the boundedness
of Toeplitz-operators on A₁ include some logarithmic
factors in a form or another. Here, we show that bounded symbols which belong to
the hyperbolic logarithmic BMO-space induce
bounded Toeplitz-operators A₁→A₁. We also
prove corresponding compactness and Fredholmness results and provide
an index formula in terms of the winding number of the symbol.
Back to main page .
|