Main page
Department of Mathematics and Statistics
of University of Helsinki
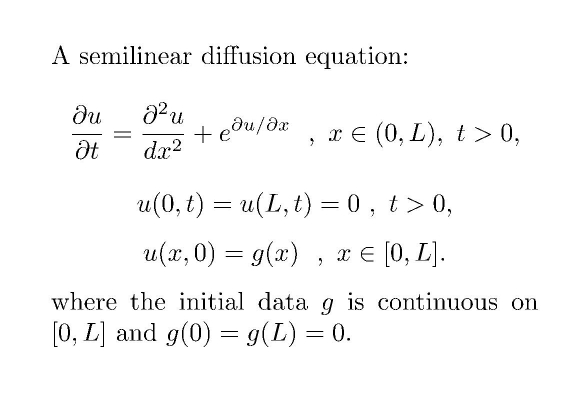
Formula 1. A semilinear equation with gradient blow-up in finite time.

Figure 1. The Bratislava Castle.
|
This research project started during the FMS International Visitor
Program "Nonlinear parabolic problems" in Autumn 2005.
SOME RECENT ARTICLES
-
M.Fila, J.Taskinen, M.Winkler:
Convergence to a singular steady state of
a parabolic equation with gradient
blow-up.
Appl.Math. Letters. 20 (2007), 578-582.
It is known (from a paper by Ph.Souplet and J.L.Vazquez, 2006)
that the semilinear initial-boundary problem presented in Formula 1
has a bounded solution u, global in time, which however ceases
to be a global classical solution: there exists a T, 0 < T < ∞ ,
such that first derivative u', or gradient, of u blows up at
the time T.
The blow-up occurs spatially at one end of the interval.
On the other hand the equation has a singular steady state
v(x) = -x ln x + x + C. Generalizing the solution u for times
t > T, a result from the above citation shows that u →
v as
t → ∞. In this paper we determine the rate of this convergence:
we show that || u -v || ≤ exp( - (λ - ε) t )
where λ is the expect convergence exponent coming from a
linearization of the problem. (It comes from the first zero of
the 0th Bessel function.)
Back to main page .
|