Main page
Department of Mathematics and Statistics
of University of Helsinki
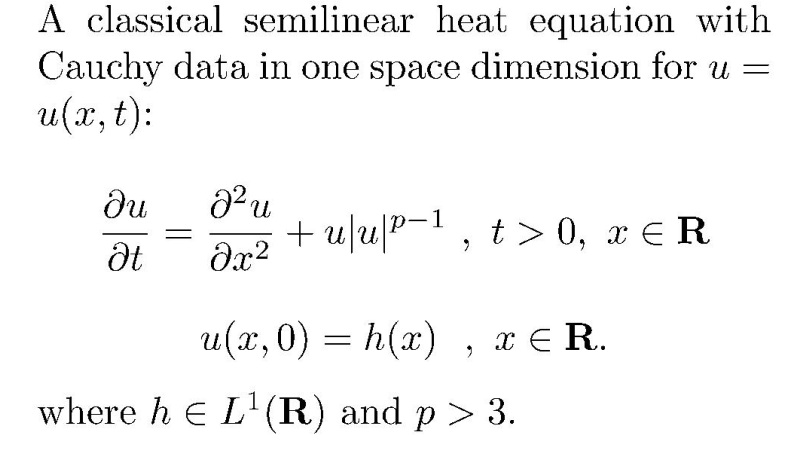
Formula 1. A classical semilinear Cauchy problem.

|
The new result on the linear case is based on abstract functional analytic methods.
The study of the long-time asymptotic behaviour
of semilinear parabolic Cauchy-problems is based on explicit integration
techniques together with weighted sup-norm estimates, applied to the
estimation of nonlinear terms in the conventional integral equation
(obtained form Duhamel's principle).
SOME RECENT ARTICLES
- J.Bonet, W.Lusky, J.Taskinen:
Schauder basis and decay rate of the heat equation.
Submitted.
We consider the (very!) classical linear heat or diffusion equation with integrable Cauchy data g in the
Eulidean space R^n. For example if the space dimension n is one, the explicit solution formula implies the
large time t decay rate t^{-1/2}, and it is known that if for example the integral of g
over the real line vanishes, then the decay rate is faster, t^{-1}. Vanishing higher iterated integrals
imply faster decay rates. In this paper we consider initial data in weighted L^p(R^n)-spaces,
where 1 < p < \infty and the
weight is fast growing at the infinity. Our result says that given arbirary natural number N, the space
can be decomposed to two components X and Y such that X is finite dimensional and if the initial data
belongs to Y, then the corresponding solution of the heat equation decays at least at the rate
t^{-N}.
The above mentioned decomposition can be done by perturbing any Schauder basis of the initial data space.
The case of p=1 is also considered, with a bit different methods.
Here is a
preprint.
-
Long time asymptotics of sub-threshold solutions of a semilinear
Cauchy problem.
Diff.Eq.Appl. 3,2 (2011), 279-297.
Restricting to the case of positive initial data (and thus solution), we
relax the assumption on the smallness of initial data in the paper below.
All sub-threshold data h can be treated at least if h is
an even function. Moreover, in addition to p ≥ 4 we also
consider the cases 3 < p < 4. Here, the perturbation term of the
solution is shown to be of the order t^(2-p)/2 instead of
1/t.
Recently, a related paper was published by Ishige, Ishiwata and Kawakami,
Indiana Univ. Math. J. (2009).
Preprint
-
Aymptotical behaviour of a class of semilinear diffusion
equations.
J.Evol.Equations 7,3 (2007), 429-447.
We show that for small initial data, the unique solution of the
semilinear Cauchy-problem as in Formula 1 behaves like the Gaussian
solution of the linear heat equation (which is of order 1/√t for
t → ∞ in space dimension 1) plus a perturbation, which is of order
1/t
only.
The result is obtained as follows. Using the Duhamel principle we
write the standard integral equation corresponding to the Cauchy
problem. Using tricky integrations by parts, several terms are
obtained. It turns out possible to process and sort them such that
those with order 1/√t behaviour are exactly of Gaussian heat
solution type, and the rest of the terms are only of order 1/t -type.
The estimation techniques for the perturbative terms emerge from the
Cahn-Hilliard paper (2005). We use weighted sup-norms for these estimates.
We restrict the considerations to one space dimension due to inessential
technical complications. However, the method applies to very general types
of nonlinearities (examples are given in the paper) as long as the
nonlinearity is of polynomial nature and of polynomial order at least 4.
Besides higher dimensions, the adoptation of this "explicit" method
to more general parabolic equations remains as an interesting challenge.
Back to main page .
|