Main page
Department of Mathematics and Statistics
of University of Helsinki

Formula 1. Bergman projections.
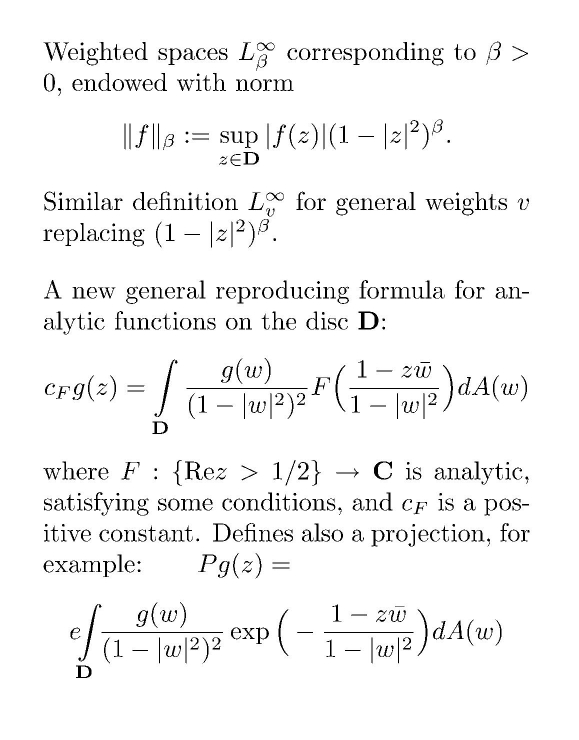
Formula 2. A new reprocuding formula.
|
Let Ω be a complex domain (in one or,
respectively, N ≥ 2
dimensions).
Bergman space Ap = Ap(Ω)
with 1 ≤ p < ∞
is the closed
subspace of Lp consisting of analytic functions.
The Lp -space is defined
with the normalized 2-dimensional, respectively,
2N-dimensional,
Lebesgue measure dA.
Bergman-projection is the orthogonal projection from L2
onto A2. In the case of the unit disc or a simply
connected
domain in one dimension there is an integral formula for the projection, see
Formula 1. If the Lp -norm is endowed with a weight
which is
the αth power of the boundary distance, one can still define the
Bergman projection of order α analogously.
In the unweighted case, the Bergman projection is not bounded
with respect to the sup norm (p= ∞). The situation is different
in the weighted cases like in Formula 2. Papers mentioned on this
page contain studies related to weighted sup-norms.
SOME RECENT ARTICLES
-
P.Erkkilä, J.Taskinen:
Sup-norm estimates for Bergman projections on
regulated domains.
Math.Scand. 102, 1 (2008), 111-130.
This work is an adoptation of the paper in Ann.Acad.Sci.Fenn (2003)
to the case p = ∞. We consider simply connnected
regulated domains and the general Bergman projections
(see Formula 1) on them. We give sufficient and necessary conditions
for their boundedness in terms
of the largest or smallest angle in the boundary curve of the domain
Ω. See the citation for more details on regulated domains.
-
J.Bonet, M.Engliš, J.Taskinen:
Weighted L∞-estimates for Bergman projections.
Studia Math.171,1 (2005), 67-92.
This paper contains three subtopics. First, we prove a new
reproducing formula (see Formula 2); it contains a general analytic
function which comes from a systematic developement of the idea of
forming analytic functions of polynomials of order α appearing
in projections in Formula 1. We show that the projection with
exponential kernel (see Formula 2) is bounded simultaneuosly for all
weighted spaces with arbitrary β.
Second, we give a partial generalization to p = ∞ of
Bekolle's Studia Math. (1982)
paper, where he gives Muckenhoupt-type conditions for weights, characterizing
boundedness of Bergman projections on weighted
Lp-spaces.
The third part contains a related consideration to solve positively
the topological subspace problem for weighted inductive limits.
The weight families under consideration satisfy logarithmic growth conditions.
-
M.Engliš, T.Hänninen, J.Taskinen:
Minimal L∞-type spaces on strictly pseudoconvex domains
on which the Bergman projection is continuous.
Houston J. Math. 32,1 (2006).
This is a nontrivial generalization of the paper below to the setting
of strictly pseudoconvex domains in higher dimensions.
-
J.Taskinen:
On the continuity of the Bergman and Szegö
projections.
Houston J. Math. 30,1 (2004), 171-190.
Motivated by the problem that the Bergman projection is not bounded
with the unweighted sup-norm, we introduce the spaces H, analytic,
and L, measurable, functions on the disc, which grow
at the boundary at most like a power of logarithm of the boundary distance.
The spaces are inductive limits, and H is a closed subspace of
L. Both of them are algebras (this property is missed
by the hyperbolic BMO space and the Bloch space). Finally, the Bergman
projection is a continuous operator from L onto H.
The corresponding space of harmonic functions and the Szegö projection also
fit into this theory in the natural way.
Back to main page .
|