Article
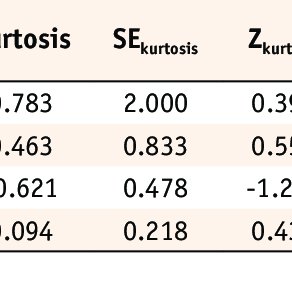
Statistical notes for clinical researchers: Assessing normal distribution (2) using skewness and kurtosis
  • February 2013
  • DOI:
  • 10.5395/rde.2013.38.1.52
Ana Adan
Diego Forero
Sabine Wilhelm
271
0 new
Recommendations
0
0 new
667
29 new
Followers
Patricia Baratta
1
0 new
ArticleStatistical notes for clinical researchers: Assessing normal distribution (2) using skewness and kurtosis
Download
Recommend this work
Get updates
Share in a message
Discuss this article with your peers
Abstract and figures
As discussed in the previous statistical notes, although many statistical methods have been proposed to test normality of data in various ways, there is no current gold standard method. The eyeball test may be useful for medium to large sized (e.g., n > 50) samples, however may not useful for small samples. The formal normality tests including Shapiro-Wilk test and Kolmogorov-Smirnov test may be used from small to medium sized samples (e.g., n < 300), but may be unreliable for large samples. Moreover we may be confused because ‘eyeball test’ and ‘formal normality test’ may show incompatible results for the same data. To resolve the problem, another method of assessing normality using skewness and kurtosis of the distribution may be used, which may be relatively correct in both small samples and large samples. 1) Skewness and kurtosis Skewness is a measure of the asymmetry and kurtosis is a measure of ’peakedness’ of a distribution. Most statistical packages give you values of skewness and kurtosis as well as their standard errors.
… 
Figure content uploaded by Hae-Young Kim
Author content
rde-38-52-normal-dist.pdf
Content uploaded by Hae-Young Kim
Author content
page 1
©Copyrights 2013. The Korean Academy of Conservative Dentistry.
52
This is an Open Access article distributed under the terms of the Creative Commons Attribution Non-Commercial License (http://creativecommons.org/licenses/
by-nc/3.0) which permits unrestricted non-commercial use, distribution, and reproduction in any medium, provided the original work is properly cited.
Statistical notes for clinical researchers: assessing
normal distribution (2) using skewness and kurtosis
As discussed in the previous statistical notes, although many statistical methods
have been proposed to test normality of data in various ways, there is no current
gold standard method.
The eyeball test
may be useful for medium to large sized
(e.g.,
n
> 50) samples, however may not useful for small samples.
The formal
normality tests
including Shapiro-Wilk test and Kolmogorov-Smirnov test may be
used from small to medium sized samples (e.g.,
n
< 300), but may be unreliable
for large samples. Moreover we may be confused because ‘eyeball test’ and ‘formal
normality test’ may show incompatible results for the same data. To resolve the
problem, another method of assessing normality using skewness and kurtosis of the
distribution may be used, which may be relatively correct in both small samples and
large samples.
1) Skewness and kurtosis
Skewness is a measure of the asymmetry and kurtosis is a measure of ’peakedness’ of
a distribution. Most statistical packages give you values of skewness and kurtosis as
well as their standard errors.
In SPSS you can find information needed under the following menu:
Analysis – Descriptive Statistics – Explore
Hae-Young Kim
Department of Dental Laboratory
Science & Engineering, Korea
University College of Health
Science, Seoul, Korea
*Correspondence to
Hae-Young Kim, DDS, PhD.
Associate Professor,
Department of Dental Laboratory
Science & Engineering, Korea
University College of Health
Science, San 1 Jeongneung 3-dong,
Seongbuk-gu, Seoul, Korea 136-703
TEL, +82-2-940-2845; FAX, +82-2-
909-3502, E-mail, kimhaey@korea.
ac.kr
Open lecture on statistics
ISSN 2234-7658 (print) / ISSN 2234-7666 (online)
http://dx.doi.org/10.5395/rde.2013.38.1.52
Skewness is a measure of the asymmetry of the distribution of a variable. The skew
value of a normal distribution is zero, usually implying symmetric distribution. A
positive skew value indicates that the tail on the right side of the distribution is
longer than the left side and the bulk of the values lie to the left of the mean.
In contrast, a negative skew value indicates that the tail on the left side of the
distribution is longer than the right side and the bulk of the values lie to the right
page 2
53
www.rde.ac
of the mean. West
et al
. (1996) proposed a reference of
substantial departure from normality as an absolute skew
value > 2.
1
Kurtosis is a measure of the peakedness of a distribution.
The original kurtosis value is sometimes called
kurtosis
(proper)
and West
et al
. (1996) proposed a reference
of substantial departure from normality as an absolute
kurtosis (proper) value > 7.
1
For some practical reasons,
most statistical packages such as SPSS provide
‘excess’
kurtosis
obtained by subtracting 3 from the kurtosis
(proper). The excess kurtosis should be zero for a
perfectly normal distribution. Distributions with positive
excess kurtosis are called leptokurtic distribution meaning
high peak, and distributions with negative excess kurtosis
are called platykurtic distribution meaning flat-topped
curve.
2) Normality test using skewness and kurtosis
A z-test is applied for normality test using skewness and
kurtosis. A z-score could be obtained by dividing the
skew values or excess kurtosis by their standard errors.
Z =
Skew value
, Z =
Excess kurtosis
SE
skewness
SE
excess kurtosis
As the standard errors get smaller when the sample
size increases, z-tests under null hypothesis of normal
distribution tend to be easily rejected in large samples
with distribution which may not substantially differ
from normality, while in small samples null hypothesis
of normality tends to be more easily accepted than
necessary. Therefore, critical values for rejecting the null
hypothesis need to be different according to the sample
size as follows:
1. For small samples (
n
< 50), if absolute z-scores for
either skewness or kurtosis are larger than 1.96, which
corresponds with a alpha level 0.05, then reject the
null hypothesis and conclude the distribution of the
sample is non-normal.
2. For medium-sized samples (50 <
n
< 300), reject the
null hypothesis at absolute z-value over 3.29, which
corresponds with a alpha level 0.05, and conclude the
distribution of the sample is non-normal.
3. For sample sizes greater than 300, depend on the
histograms and the absolute values of skewness
and kurtosis without considering z-values. Either
an absolute skew value larger than 2 or an absolute
kurtosis (proper) larger than 7 may be used as reference
values for determining substantial non-normality.
Referring to Table 1 and Figure 1, we could conclude all
the data seem to satisfy the assumption of normality
Table 1.
Skewness, kurtosis and normality tests for a characteristic of interests in various sizes of samples
Sample size
Skewness
SE
skewnwss
Z
skewness
Kurtosis
SE
kurtosis
Z
kurtosis
Kolmogorov-Smirnov*
Shapiro-Wilk
(n)
Statistics
p
-value
Statistics
p
-value
5
-0.971
0.913
-1.064
0.783
2.000
0.392
0.191
0.200
0.948
0.721
30
0.285
0.427
0.667
0.463
0.833
0.556
0.068
0.200
0.988
0.976
100
0.105
0.241
0.436
-0.621
0.478
-1.299
0.076
0.167
0.983
0.216
500
-0.251
0.109
-2.303
0.094
0.218
0.431
0.044
0.020
0.993
0.029
*Lilliefors significance correct
2.0
1.5
1.0
0.5
0.0
n
= 5
10
8
6
4
2
0
n
= 30
20
15
10
05
0.0
n
= 100
60
50
40
30
20
10
0
n
= 500
Figure 1.
Histograms of a characteristic of interests in various sizes of samples.
page 3
54
www.rde.ac
despite that the histogram of the smallest-sized sample
doesn’t appear as a symmetrical bell shape and the formal
normality tests for the largest-sized sample were rejected
against the normality null hypothesis.
3) How strict is the assumption of normality?
Though the humble
t
test (assuming equal variances)
and analysis of variance (ANOVA) with balanced sample
sizes are said to be ‘robust‘ to moderate departure
from normality, generally it is not preferable to rely
on the feature and to omit data evaluation procedure.
A combination of visual inspection, assessment using
skewness and kurtosis, and formal normality tests can
be used to assess whether assumption of normality is
acceptable or not. When we consider the data show
substantial departure from normality, we may either
transform the data, e.g., transformation by taking
logarithms, or select a nonparametric method such that
normality assumption is not required.
Reference
1.
West SG, Finch JF, Curran PJ. Structural equation models
with nonnormal variables: problems and remedies. In
RH Hoyle (Ed.). Structural equation modeling: Concepts,
issues and applications. Newbery Park, CA: Sage; 1995.
p56-75.
Kim HY
Mark as interesting Comment Delete highlight
Similar research
On measuring skewness and kurtosis
Article
  • May 2009
  • Quality and Quantity
The paper considers some properties of measures of asymmetry and peakedness of one dimensional distributions. It points to some misconceptions of the first and the second Pearson coefficients, the measures of asymetry and shape, that frequently occur in introductory textbooks. Also it presents different ways for obtaining the estimated values for the coefficients of skewness and kurtosis and statistical tests which include them. KeywordsSkewness-Kurtosis-Estimates of moments
Statistical notes for clinical researchers: simple linear regression 2 – evaluation of regression line
Article
Full-text available
  • Aug 2018
Skewness and kurtosis of net baryon-number distributions at small values of the baryon chemical potential
Article
Full-text available
  • Oct 2017
We present results for the ratios of mean (MB), variance (σB2), skewness (SB) and kurtosis (κB) of net baryon-number fluctuations obtained in lattice QCD calculations with physical values of light and strange quark masses. Using next-to-leading order Taylor expansions in baryon chemical potential we find that qualitative features of these ratios closely resemble the corresponding experimentally measured cumulant ratios of net proton-number fluctuations for beam energies down to sNN≥19.6 GeV. We show that the difference in cumulant ratios for the mean net baryon-number, MB/σB2=χ1B(T,μB)/χ2B(T,μB), and the normalized skewness, SBσB=χ3B(T,μB)/χ2B(T,μB), naturally arises in QCD thermodynamics. Moreover, we establish a close relation between skewness and kurtosis ratios, SBσB3/MB=χ3B(T,μB)/χ1B(T,μB) and κBσB2=χ4B(T,μB)/χ2B(T,μB), valid at small values of the baryon chemical potential.
Statistical notes for clinical researchers: simple linear regression 1 – basic concepts
Article
Full-text available
  • Apr 2018
Statistical notes for clinical researchers: logistic regression
Article
Full-text available
  • Nov 2017